• View
• Print
• Backlinks

Rolling the Box

Think of the box sitting on the {$x-y$} plane on top of a line from the origin at an angle of {$45^\circ$}.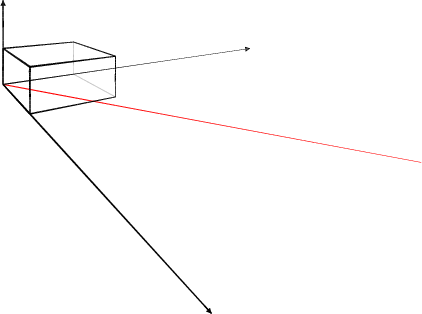

When the path reaches an edge,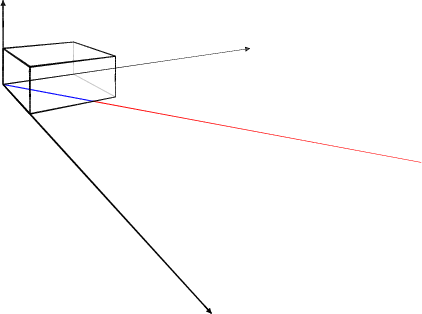

think of the box as rolling over.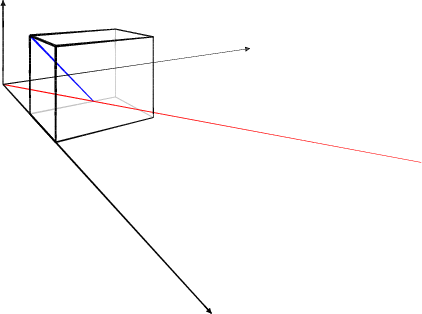

Then the path moves on to the next edge,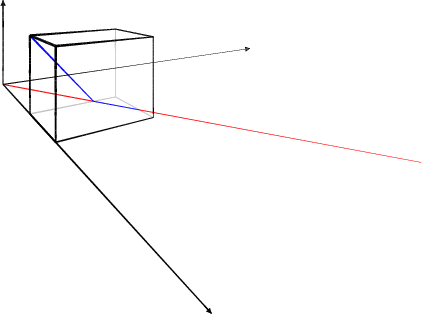

and the box rolls again, always remaining on the red line.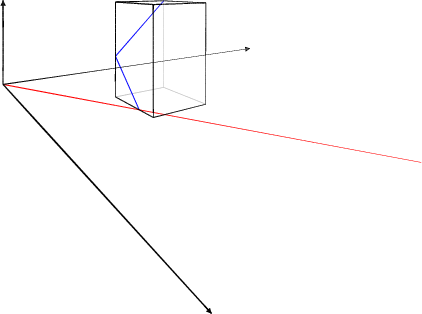

And so on. The pattern left on the plane,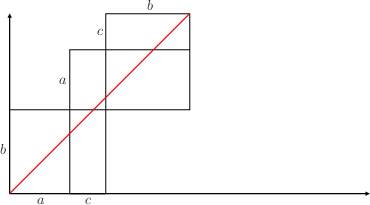

is useful for understanding how it that in this case the path leads back to the starting point.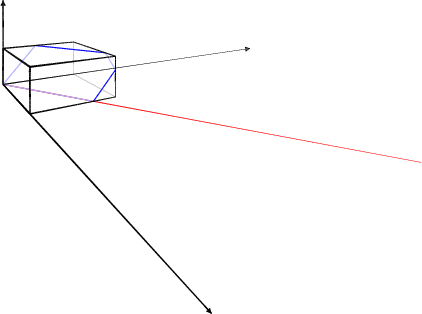

There is yet another way to see the geodesic on a flat plane. This method uses a three-colorable map.